8 x 10
First row:
A remarkable solution with two congruent halves. Every piece has piece of the same shape in the same location in the opposite half.
A second half-and-half solution: each half has two of each shape.The other two solutions are nearly divided in half along the long dimension.
Second row:
The two solutions could have their red and yellow pieces interchanged OR just the red and yellow squares, since each adjoins only blue and green, OR just the other four pairs of pieces, since they form a chain which adjoins only blue and green also.Third row:
The next two have just the red and yellow T's and Z's swapped.The last two have just the red and blue bars swapped.
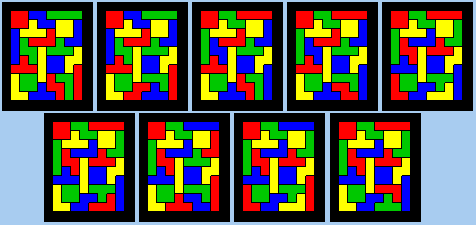
Fourth and fifth rows:
Show nine solutions with many pieces in common and just a minority swapped in various ways.9 x 9
Those with black frames come from the 90 solutions which have symmetry: with a 180-degree rotation and swapping of colors, the solution remains the same. The ones with gray frames (and an asterisk) do not have this property.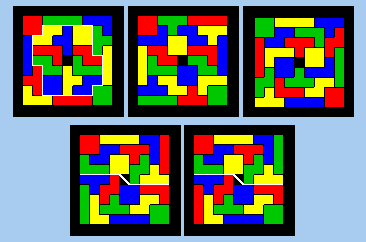
- A ring of pieces and a center. Alternating strips of red/green and blue/yellow, aligned roughly from upper right to lower left.
- A ring of blue and yellow, with red/green stripes above, below, and in the center.
- Two "S"s, intersecting in the center. Blue/yellow as an S, red/green
resembling a "u n".
Second row:
- Two solutions which divide in half.
They differ by having the red and green bars swapped.
First row:
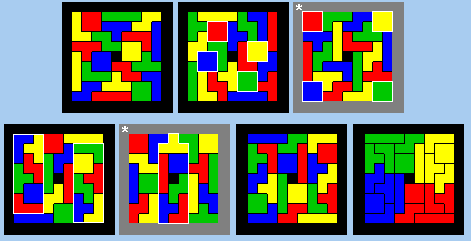
- Alternating upper-right/lower-left strips, resembling a staircase.
- All the square pieces in the interior.
- The square pieces in the four corners.
The shape is symmetrical but the coloring is not. If the green and blue squares were swapped, the coloring would be symmetrical, too.Fourth row:
- Three 3x9 near-rectangles, with two actual 3x8 rectangles.
- "Absolut Tetrominoes".
- The last two have the same shape. By swapping some same-shape pieces we get a solution grouped by color. Surely there are more ways to explore these arrangements, but their investigation awaits another day.